When discussing statistics, mathematics, or even everyday situations, the terms "mean" and "average" often come into play. But what do they truly signify, and how do they differ? While they are frequently used interchangeably, understanding the subtle distinctions between "mean average vs average" is crucial for accurate data interpretation. These terms are fundamental in various fields, from education to economics, and knowing their differences can significantly impact analysis and decision-making.
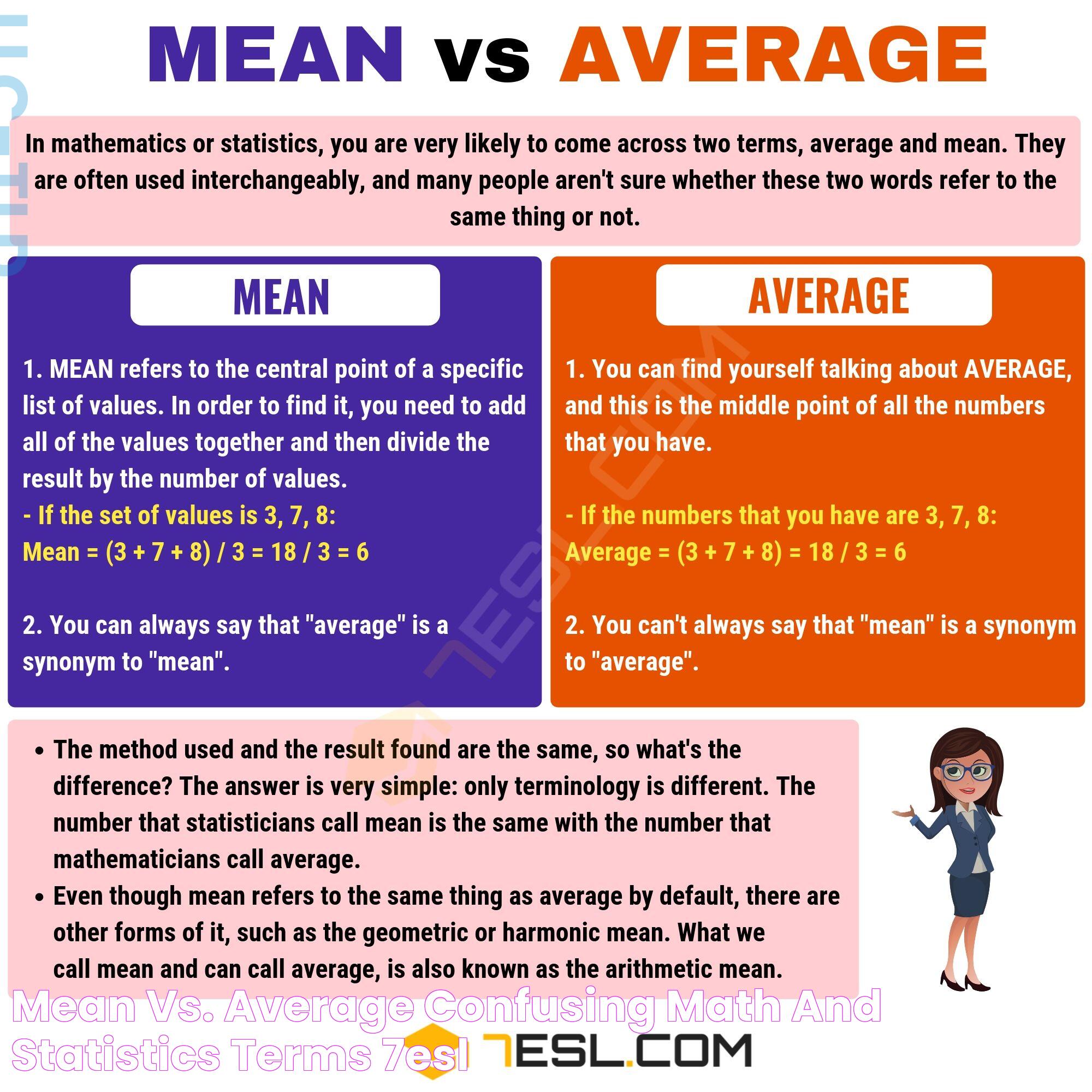
At its core, the term "average" refers to a central or typical value within a set of numbers. It's a broad concept that encompasses different types of averages, including mean, median, and mode. In contrast, "mean" is a specific type of average, often referred to as the "arithmetic mean." It is calculated by adding all numbers in a dataset and then dividing by the count of numbers. This distinction is not merely academic but has practical implications in data analysis and interpretation.
As we delve deeper into the topic, this guide will explore the nuances of "mean average vs average," examining their definitions, differences, and real-world applications. We'll also discuss common misconceptions, provide illustrative examples, and highlight the importance of choosing the right form of average in various contexts. By the end of this article, you'll have a comprehensive understanding of these concepts and be better equipped to apply them effectively in your analyses.
Read also:Mastering Loose Curl Iron Techniques For Flawless Waves
Table of Contents
- What is an Average?
- Understanding the Mean
- Mean Average vs Average: Unpacking the Differences
- Types of Averages: Beyond the Mean
- When to Use Mean vs Average?
- Real-World Applications of Mean and Average
- Common Misconceptions about Mean and Average
- Calculating the Mean: A Step-by-Step Guide
- Mean vs Median: Which is More Reliable?
- Impact of Outliers on Mean Average vs Average
- Role of Mean and Average in Statistics
- Examples of Mean and Average in Practice
- How is Average Used in Daily Life?
- Frequently Asked Questions
- Conclusion
What is an Average?
The term "average" is a broad concept used to find a central or typical value within a dataset. It is a measure of central tendency that is employed in various fields such as mathematics, statistics, and everyday life to summarize data. Averages help us understand the general trend of a dataset by providing a single value that represents the entire set.
There are three main types of averages: mean, median, and mode. Each type of average provides different insights into the dataset, and the choice of which to use depends on the specific context and nature of the data. The average you choose can significantly affect data interpretation, making it essential to understand the differences between them.
Types of Averages
- Mean: This is the most commonly used type of average. It is calculated by summing all the numbers in a dataset and then dividing by the number of values.
- Median: The median is the middle value when the numbers in a dataset are arranged in ascending or descending order. If there is an even number of observations, the median is the average of the two middle numbers.
- Mode: The mode is the number that appears most frequently in a dataset. It is possible for a dataset to have more than one mode or no mode at all.
Understanding the Mean
The mean, often referred to as the "arithmetic mean," is a specific type of average. It is calculated by adding all the values in a dataset and dividing the total by the number of values. This provides a central value that represents the dataset as a whole. The mean is widely used because it considers all data points, providing a comprehensive view of the dataset.
One of the main advantages of the mean is its simplicity and ease of calculation. It is a straightforward method that is easy to understand and apply. However, the mean can be influenced by extreme values or outliers, which can skew the results and provide a misleading representation of the dataset.
Calculating the Mean
To calculate the mean, follow these steps:
- Sum all the numbers in the dataset.
- Count the number of values in the dataset.
- Divide the total sum by the number of values.
For example, in the dataset {5, 10, 15, 20, 25}, the mean is calculated as follows:
Read also:Astrological Insights The 7th Rashi In Vedic Astrology
- Total Sum: 5 + 10 + 15 + 20 + 25 = 75
- Number of Values: 5
- Mean: 75 / 5 = 15
Mean Average vs Average: Unpacking the Differences
The terms "mean average" and "average" are often used interchangeably, but they have distinct meanings in statistical analysis. Understanding these differences is crucial for accurate data interpretation and analysis.
The term "average" is a general term that refers to a central value in a dataset. It can refer to the mean, median, or mode, depending on the context. The mean average, on the other hand, specifically refers to the arithmetic mean, which is the sum of all values divided by the number of values.
Key Differences
- Definition: The average is a broad term that includes mean, median, and mode, while the mean average specifically refers to the arithmetic mean.
- Calculation: The mean average is calculated by summing all values and dividing by the number of values, while the calculation for median and mode differs.
- Use Cases: The mean average is suitable for evenly distributed datasets, while the median is better for skewed data or datasets with outliers.
Types of Averages: Beyond the Mean
While the mean is the most commonly used average, it's important to understand the other types of averages and their applications. Each type of average provides unique insights into the dataset, and choosing the right one can significantly impact data analysis.
Median
The median is the middle value in a dataset when the numbers are arranged in order. It is a useful measure of central tendency for skewed datasets or when there are outliers, as it is not affected by extreme values.
Mode
The mode is the value that appears most frequently in a dataset. It is useful for categorical data or datasets where the most common value is of interest. Datasets can have one mode, more than one mode (bimodal or multimodal), or no mode at all.
When to Use Mean vs Average?
Choosing between the mean and other types of averages depends on the nature of the dataset and the specific analysis goals. Each type of average has its strengths and weaknesses, and understanding when to use each is crucial for accurate data interpretation.
When to Use Mean
- For datasets with evenly distributed values and no outliers.
- When all data points are equally important and need to be considered.
- For large datasets where small variations do not significantly affect the mean.
When to Use Median or Mode
- For skewed datasets or datasets with outliers, the median provides a better central value.
- For categorical data or datasets where the most common value is of interest, the mode is suitable.
- When the dataset has a few extreme values that could skew the mean.
Real-World Applications of Mean and Average
The concepts of mean and average are used extensively in various fields, from education to economics. Understanding their applications can provide valuable insights into data interpretation and decision-making.
Applications in Education
In education, averages are used to calculate students' grades, determine class performance, and analyze test scores. The mean provides an overall view of student performance, while the median can highlight the typical student's performance.
Applications in Economics
In economics, averages are used to analyze financial data, such as income levels, consumer spending, and inflation rates. The mean and median can provide insights into economic trends and help policymakers make informed decisions.
Common Misconceptions about Mean and Average
Despite their widespread use, there are several misconceptions about mean and average that can lead to inaccurate data interpretation. Understanding these misconceptions is essential for effective data analysis.
Misconception: Mean and Average are the Same
Many people use the terms "mean" and "average" interchangeably, but they have distinct meanings in statistics. While the mean is a specific type of average, the term "average" encompasses mean, median, and mode.
Misconception: Mean is Always the Best Measure
While the mean is a useful measure of central tendency, it is not always the best choice. In skewed datasets or datasets with outliers, the median or mode may provide a more accurate representation of the data.
Calculating the Mean: A Step-by-Step Guide
Calculating the mean is a straightforward process that involves summing all the values in a dataset and dividing by the number of values. This section provides a detailed step-by-step guide to calculating the mean.
Step-by-Step Guide
- Sum all the numbers in the dataset.
- Count the number of values in the dataset.
- Divide the total sum by the number of values.
For example, in the dataset {8, 12, 16, 20, 24}, the mean is calculated as follows:
- Total Sum: 8 + 12 + 16 + 20 + 24 = 80
- Number of Values: 5
- Mean: 80 / 5 = 16
Mean vs Median: Which is More Reliable?
When analyzing data, choosing between the mean and median depends on the dataset's characteristics. Each has its strengths and applications, and understanding which is more reliable can impact data interpretation.
Mean: Pros and Cons
- Pros: Considers all data points, easy to calculate, provides a comprehensive view.
- Cons: Can be skewed by outliers, may not represent typical values in skewed datasets.
Median: Pros and Cons
- Pros: Not affected by outliers, provides a central value for skewed datasets.
- Cons: Does not consider all data points, may not provide a complete view of the dataset.
Impact of Outliers on Mean Average vs Average
Outliers are extreme values in a dataset that can significantly affect the mean. Understanding the impact of outliers is crucial for accurate data analysis and interpretation.
Impact on Mean
Outliers can skew the mean, making it unrepresentative of the dataset as a whole. This is because the mean considers all data points, including extreme values, which can distort the central tendency.
Impact on Median and Mode
Outliers have little to no impact on the median and mode. The median, being the middle value, is not affected by extreme values, while the mode, representing the most frequent value, is also unaffected by outliers.
Role of Mean and Average in Statistics
The mean and average play a crucial role in statistical analysis, providing insights into data distribution and central tendency. Understanding their applications in statistics is essential for effective data interpretation.
Mean in Statistical Analysis
The mean is used to summarize data and identify trends. It is a fundamental measure in descriptive statistics and is often used in hypothesis testing, regression analysis, and other statistical methods.
Average in Statistical Analysis
Averages, including mean, median, and mode, provide a comprehensive view of data distribution. They are used to identify central trends, compare datasets, and make informed decisions based on data analysis.
Examples of Mean and Average in Practice
Understanding the practical applications of mean and average can provide valuable insights into their use in various fields. This section provides illustrative examples of mean and average in practice.
Example 1: Calculating Student Grades
In education, the mean is often used to calculate students' grades. For example, if a student has grades of 85, 90, 78, 92, and 88, the mean grade is calculated as follows:
- Total Sum: 85 + 90 + 78 + 92 + 88 = 433
- Number of Grades: 5
- Mean Grade: 433 / 5 = 86.6
Example 2: Analyzing Income Levels
In economics, the median is often used to analyze income levels, as it provides a more accurate representation of the typical income in a skewed dataset with extreme values.
How is Average Used in Daily Life?
Averages are used in various aspects of daily life, from calculating expenses to analyzing trends. Understanding their applications in everyday situations can provide valuable insights into their practical use.
Daily Life Applications
- Calculating average expenses to manage budgets.
- Analyzing average temperatures to plan activities.
- Determining average commute times for travel planning.
Frequently Asked Questions
What is the difference between mean and average?
The mean is a specific type of average calculated by summing all values and dividing by the number of values. The average is a broader term that includes mean, median, and mode.
When should I use the mean instead of the median?
The mean is suitable for evenly distributed datasets without outliers. The median is better for skewed datasets or when outliers are present.
How do outliers affect the mean average?
Outliers can skew the mean, making it unrepresentative of the dataset. The median is less affected by outliers and may be a better measure in such cases.
Can a dataset have more than one mode?
Yes, a dataset can have more than one mode (bimodal or multimodal) if multiple values appear with the same highest frequency.
What are the limitations of using the mean?
The mean can be skewed by outliers and may not represent typical values in skewed datasets. It also assumes all data points are equally important.
How is the average used in real-world scenarios?
Averages are used to summarize data, identify trends, and make informed decisions in fields such as education, economics, and everyday life.
Conclusion
In summary, understanding the differences between "mean average vs average" is essential for accurate data analysis and interpretation. While these terms are often used interchangeably, they have distinct meanings and applications in statistics and everyday life. The mean is a specific type of average that provides a comprehensive view of a dataset, while the average encompasses mean, median, and mode. By understanding these concepts and choosing the appropriate type of average, you can make informed decisions based on data analysis and gain valuable insights into various fields and real-world scenarios.